Metallic Additive Manufacturing (AM)
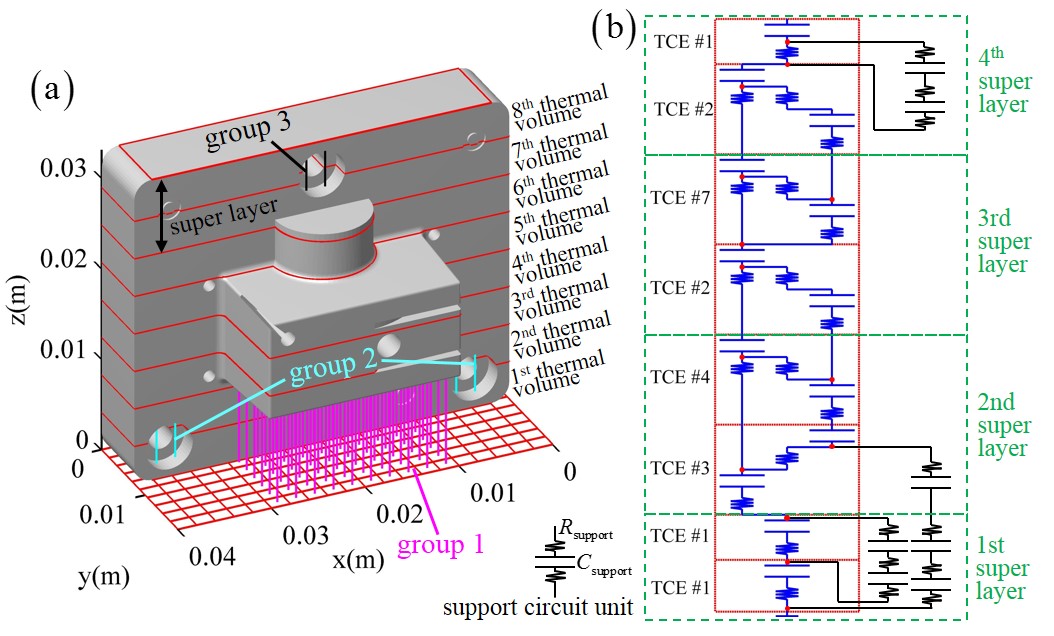
Part-scale metallic AM model: metallic AM processes, including selective laser melting (SLM), electron beam melting (EBM) and direct metal laser sintering (DMLS) can quickly build parts with complex geometry. To optimize part orientation and support structure for minimum part distortion in DMLS, fast thermal and thermo-mechanical models are developed to predict the temperature history and part distortion. For predicting the temperature history, a novel thermal circuit network (TCN) model is developed to model the heat transfer in DMLS process as shown in Fig. (a) and (b). For predicting the part distortion, a quasi-static thermo-elasto-plastic FEM model is developed by using the temperature history from the TCN model. The stresses and displacement of the part on substrate and off substrate are predicted.
Part built horizontally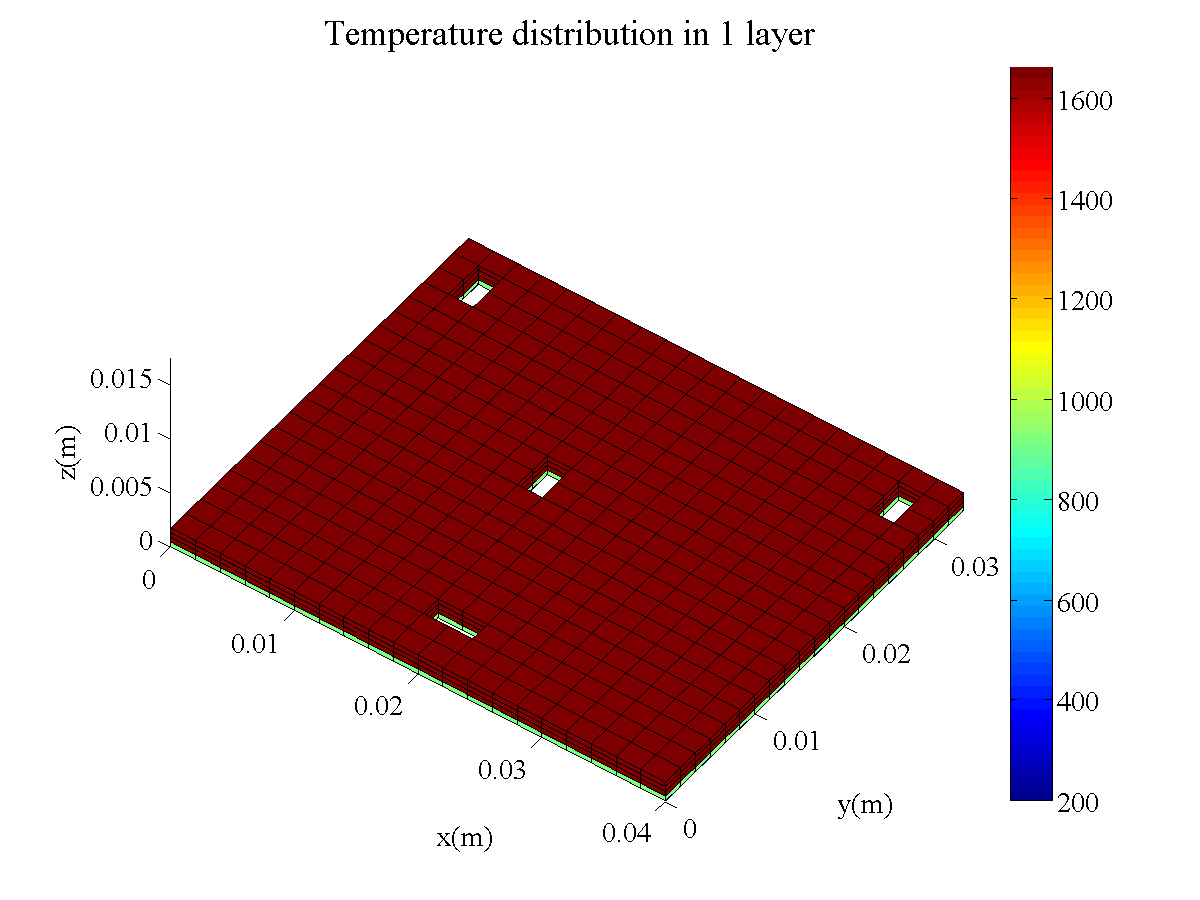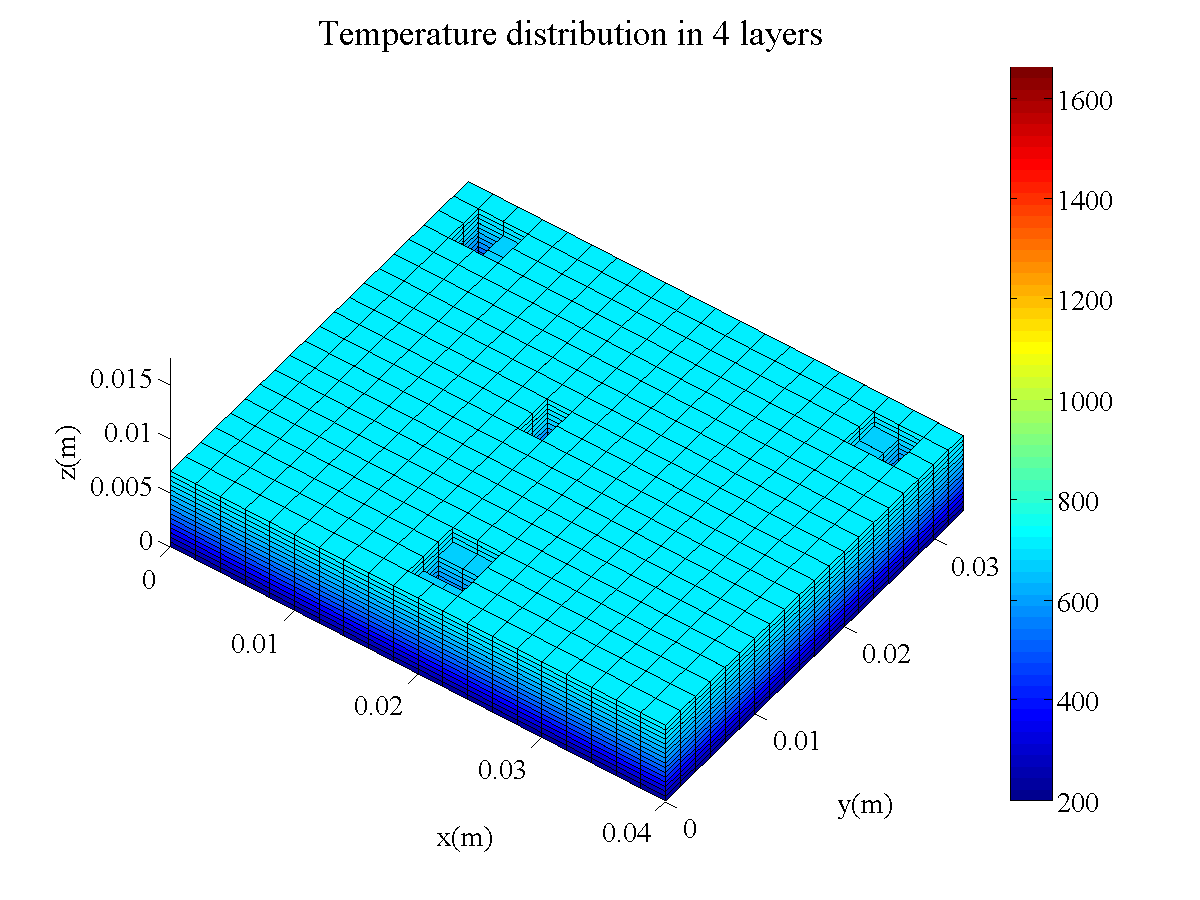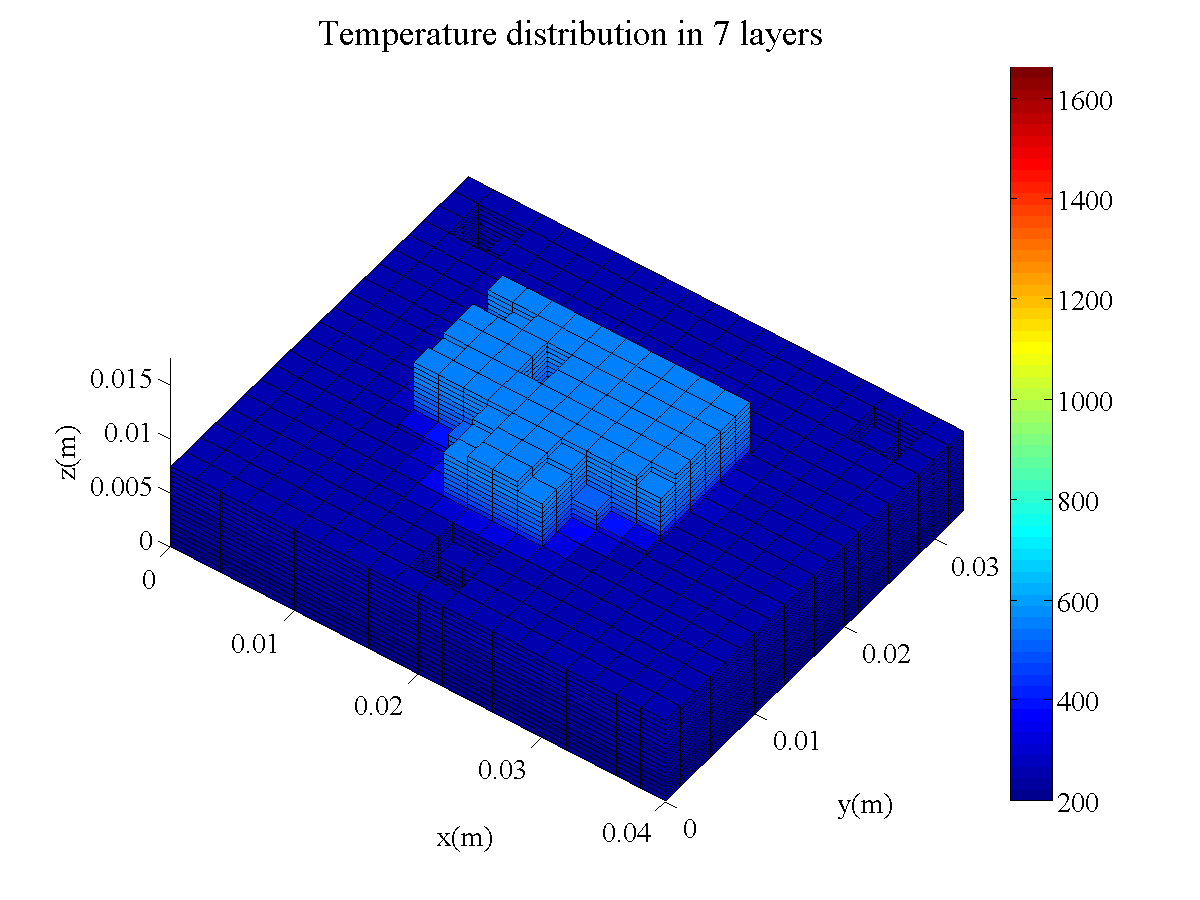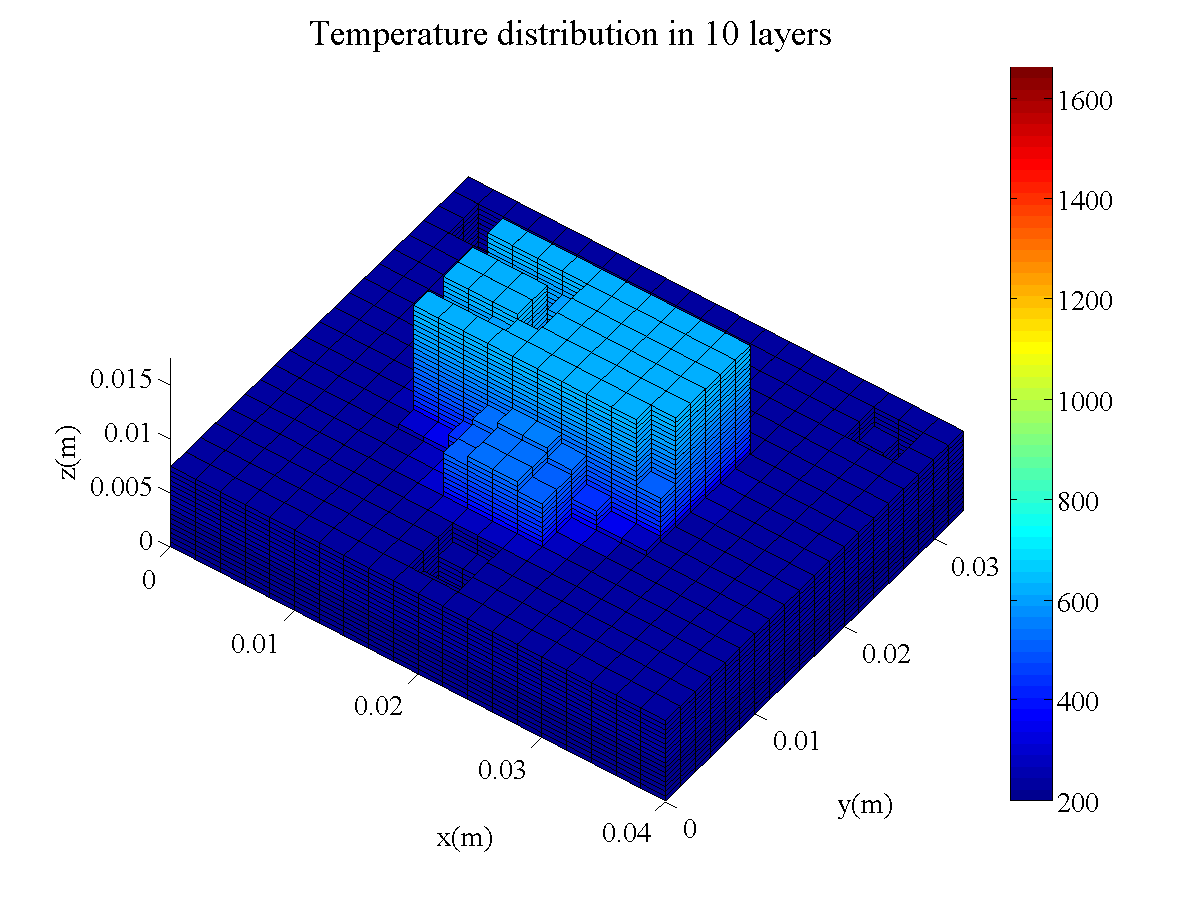 |
Part built vertically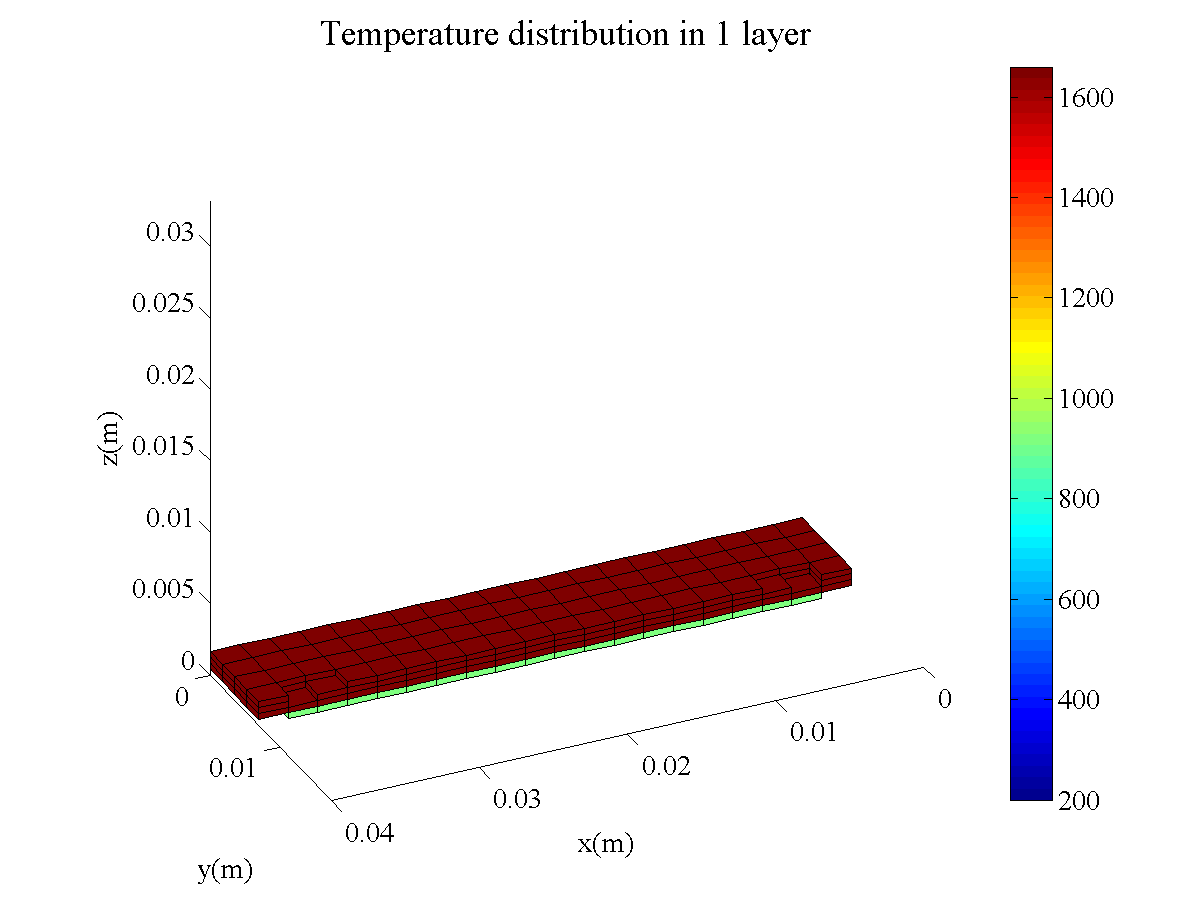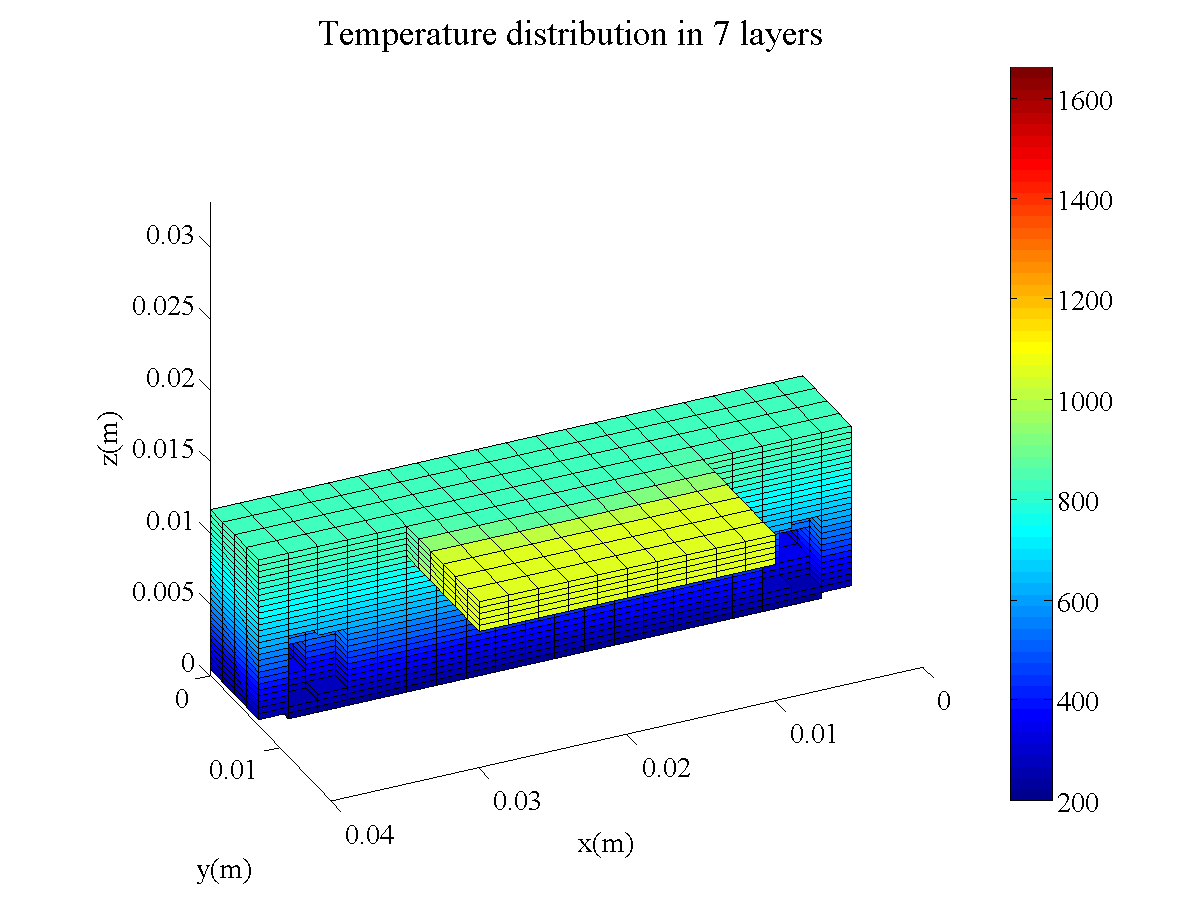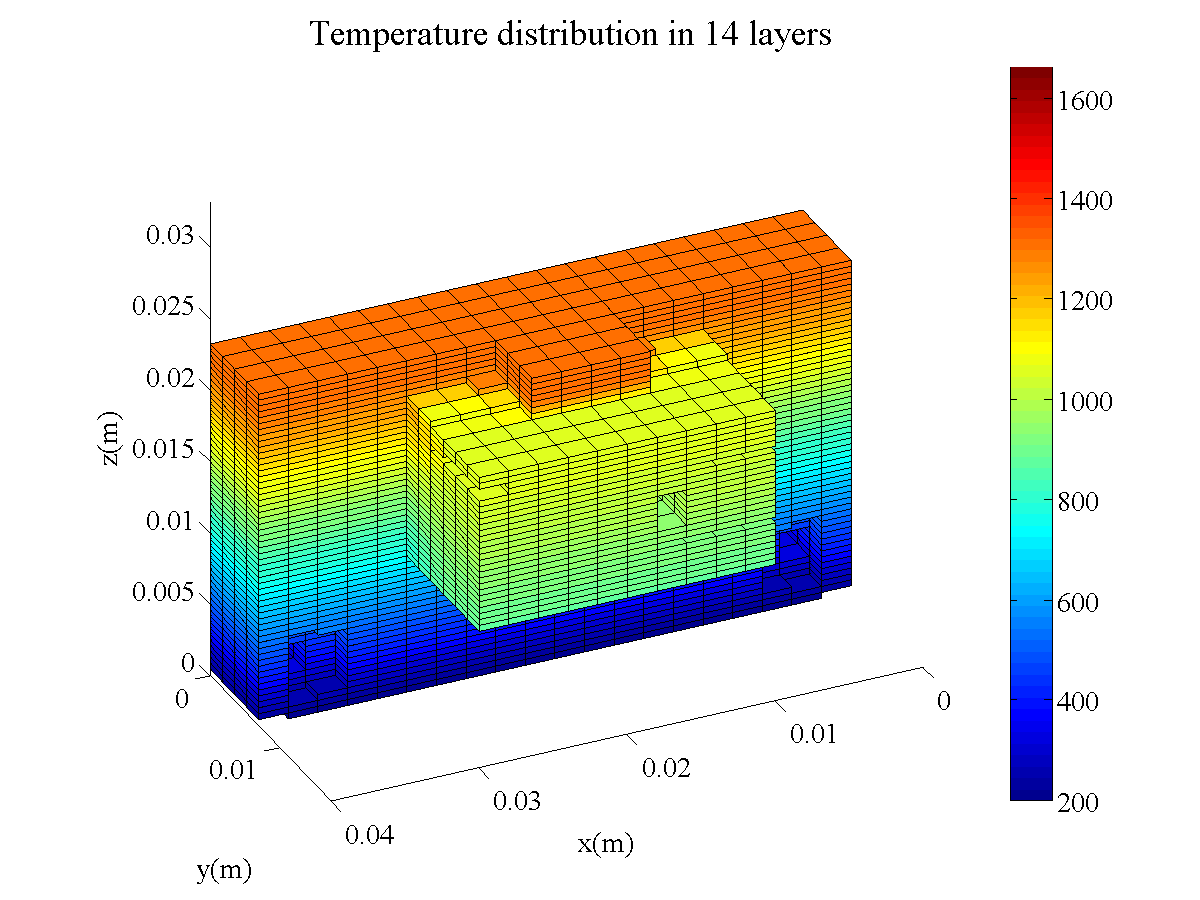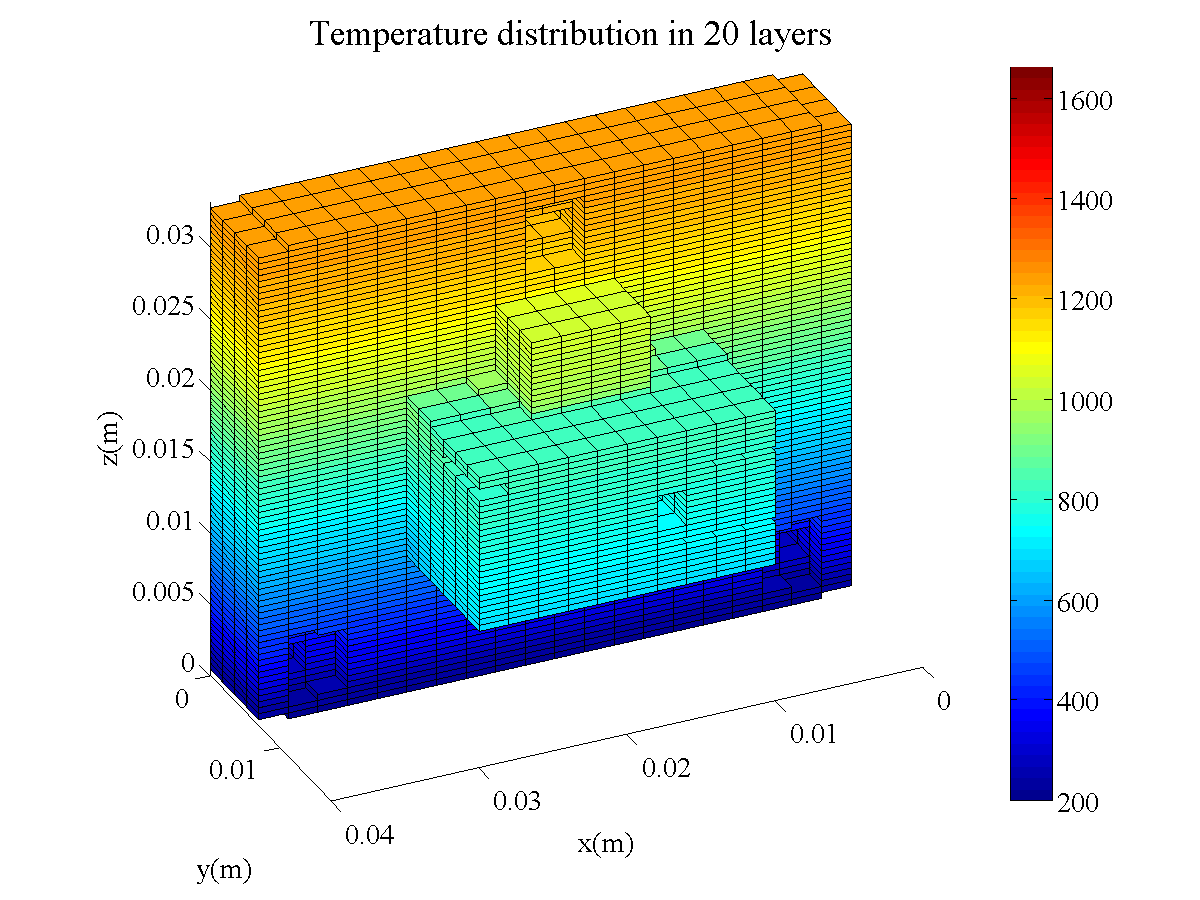 |
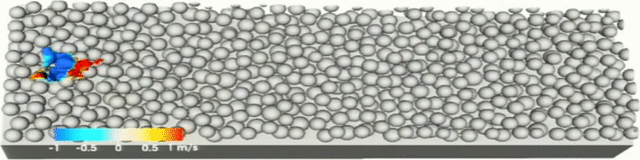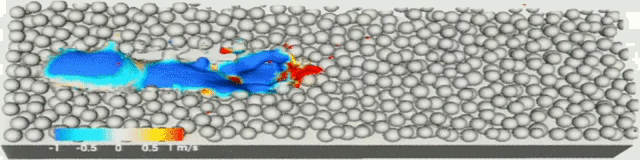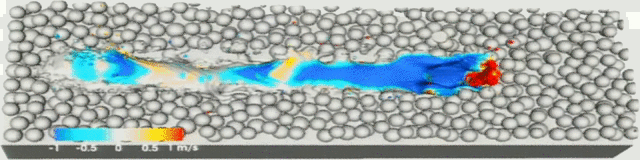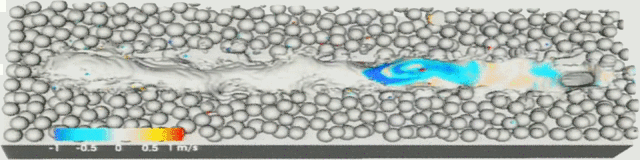
Meso-scale metallic AM model: a reliable meso-scale metallic AM model should consider laser/electron beam absorption, heat conduction into the metal powder bed, convection and radiative heat transfer into the inert gas phase, phase change of metal powders, Marangoni convection in the melt pool, balling effect due to Plateau-Rayleigh instability, the dynamic motion of metal powders and the residual stresses after manufacturing. The state-of-the-art modeling methods of AM range from traditional finite element (FE) or finite volume methods, to novel approaches such as volume of fluid method (VOF), level-set method, lattice Boltzmann method (LBM) and discrete element method (DEM). The following shows a meso-scale model developed by Lawrence Livermore National Lab and the comparison to the experiment.
Acoustic Metamaterial Design
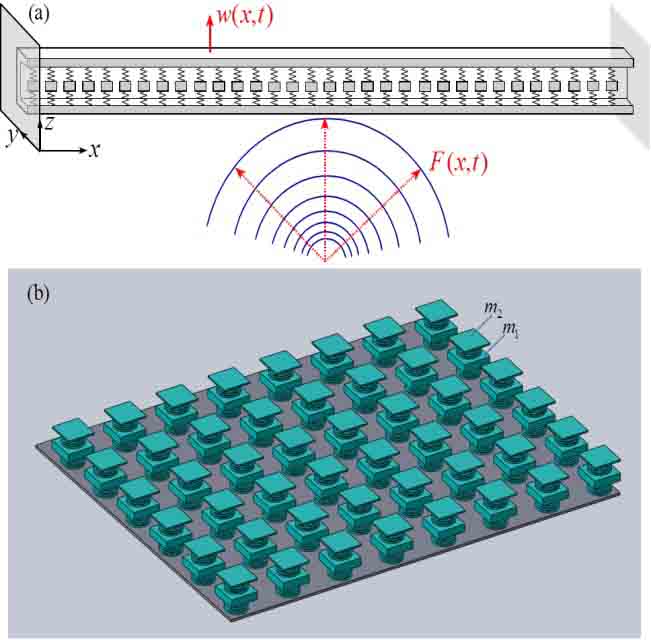
Acoustic metamaterials are intelligent material structures that can attenuate/stop or guide an elastic wave propagating along a desired path by employing the resonance of the integrated local subsystems. If elastic waves are not allowed to propagate within a successive frequency band, the frequency band is called stopband. Figure (a) shows a single-stopband acoustic metamaterial beam and Fig.(b) shows a two-stopband acoustic metamaterial plate (local subsystems denoted by m1 and m2). The two stopbands of the metamaterial plate is manifested by the dispersion relations shown in Fig. (c). Both metamaterial structures use mass-spring subsystems as vibration absorbers.
 |
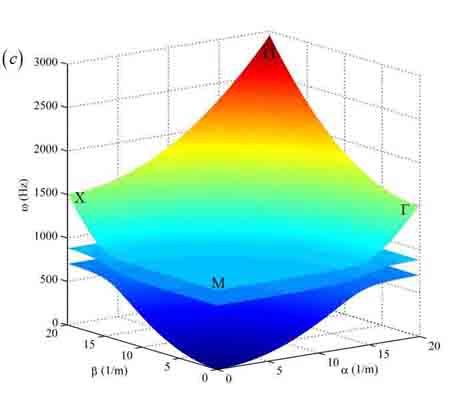 |
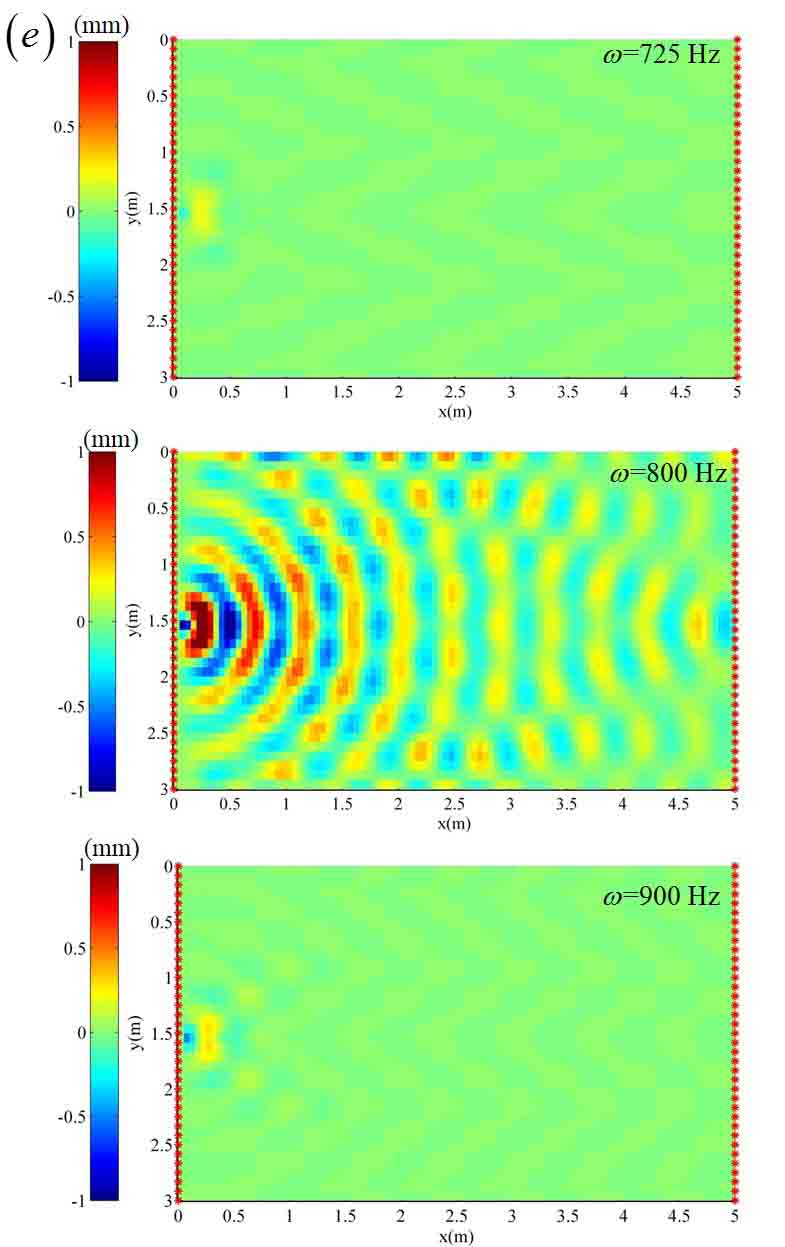
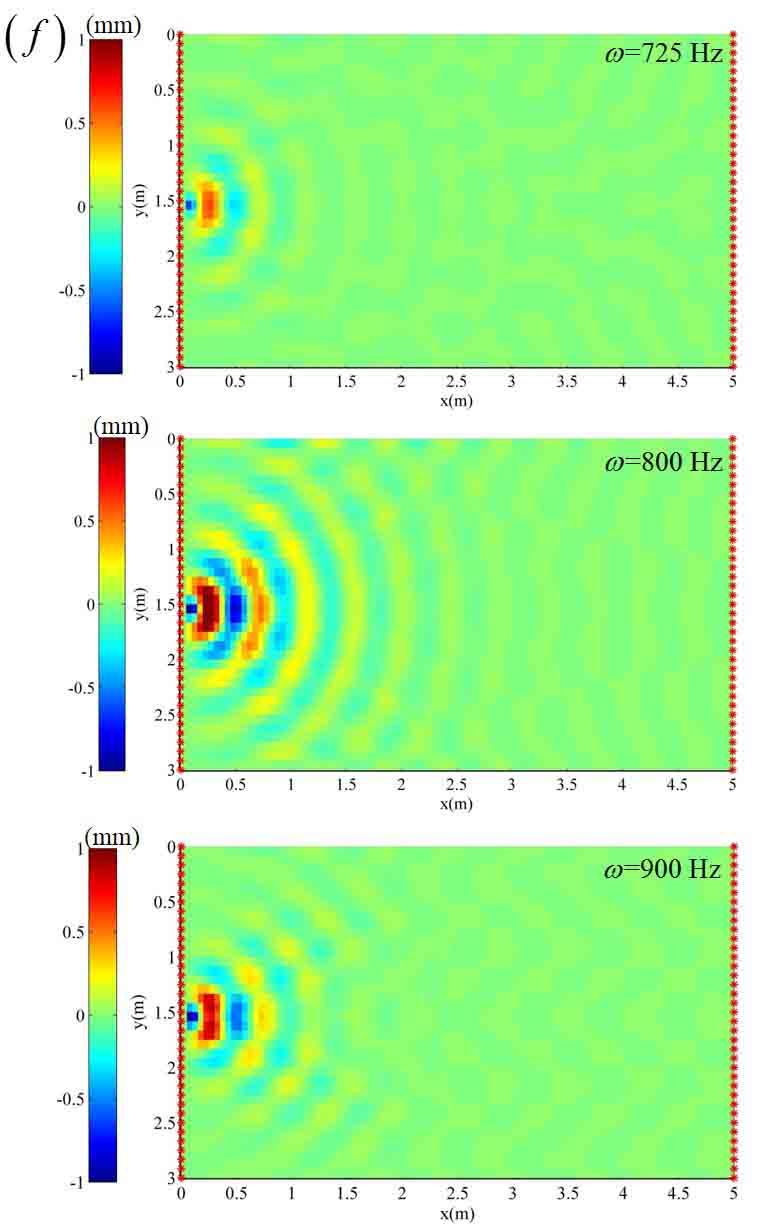
Figure (d-f) show the steady state vibration pattern of a two-stopband acoustic metamaterial plate with different damping for m2 under three different vibration frequencies (two frequencies in the two stoptands, respectively, and one in between). Each stopband's bandwidth can be increased by increasing the absorber mass and/or reducing the isotropic plate's unit cell mass. Moreover, a high damping for m2 can help combine the two stopbands into a wide one as shown in Fig. (f).
Oscillating Heat Pipes (OHPs)
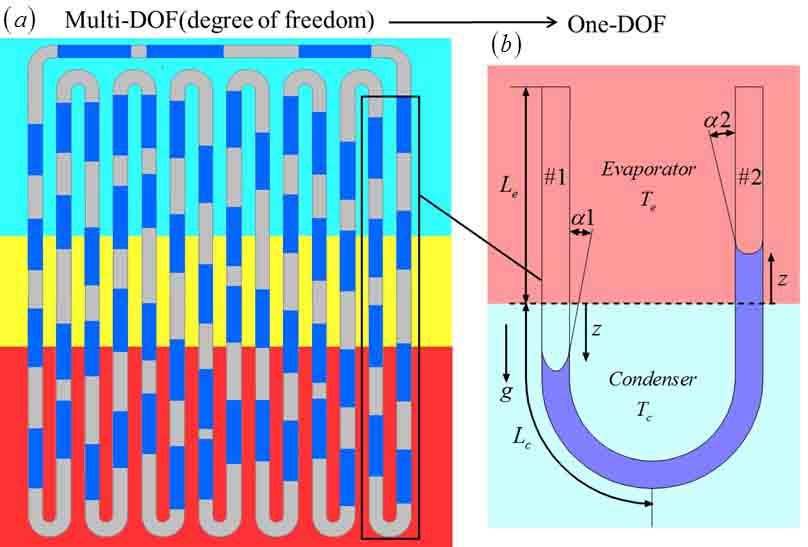
OHPs are promising heat dissipation devices for modern electronic systems. However, accurate modeling of OHPs is hard because OHPs are essentially complicated multiphysics device, which incorporates mechanical dynamics, convective heat transfer, thin film evaporation/condensation into a single problem. Figure (a) shows a typical OHP and Fig. (b) is its one-DOF working unit. The red, cyan and yellow backgrounds in Fig. (a) represent evaporator, condenser and adiabatic area, respectively. The liquid slugs in the capillary tube are in blue and the vapor slugs are in grey.
 |
The modal analysis of an OHP reveals its inherent working mechanism. The zero-frequency movement (rigid-body movement) is crucial because it initiates and sustains the two-phase flow, carrying heat continuously from the evaporator to the condenser. Although the high-frequency modes, e.g., mode #3, do not contribute to the circulation of the flow, high-frequency oscillation of liquid slugs creates high velocity/temperature gradients and significantly increases convective heat transfer between the liquid slugs and the tube wall. Besides, the trailing edge of the quick-moving liquid slugs leave thin films on the tube wall and facilitate the phase-change heat transfer. In conclusion, an appropriate combination of different modes guarantees the high heat transfer capability of an OHP.
mode #1 f=0Hz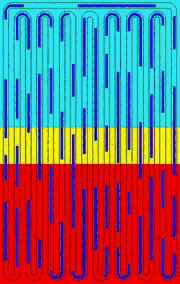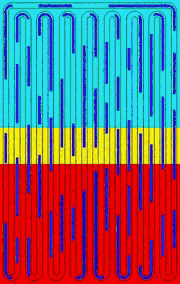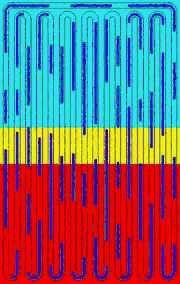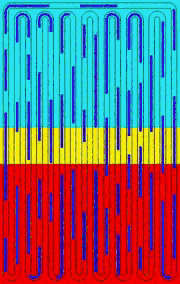 |
mode #3 f=5.03Hz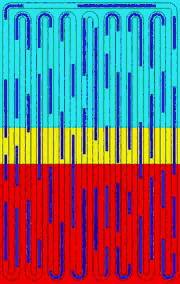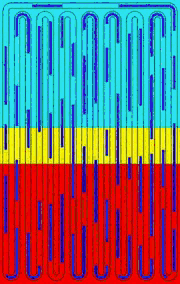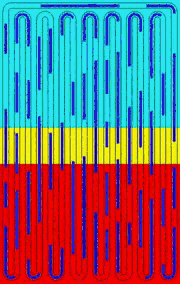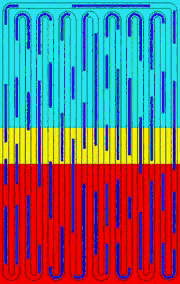 |
mode #1+#3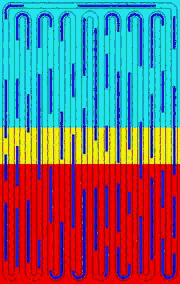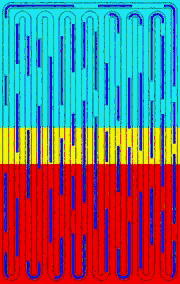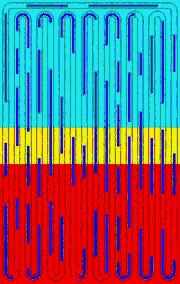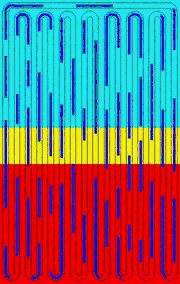 |
The heat transfer of an OHP comes from two parts, convective heat transfer and phase-change heat transfer. The phase-change heat transfer accounts for less than 10% of the total heat transfer, which is consistent with the experimental data in literatures. Despite of the low percentage of phase-change heat transfer, the high-pressure vapor generated during the phase change process provides driving force for the oscillating flow. When the external heat power increases, the phase-change heat transfer increases also, which makes the heat tranfer capability of an OHP almost proportional to the heat input.
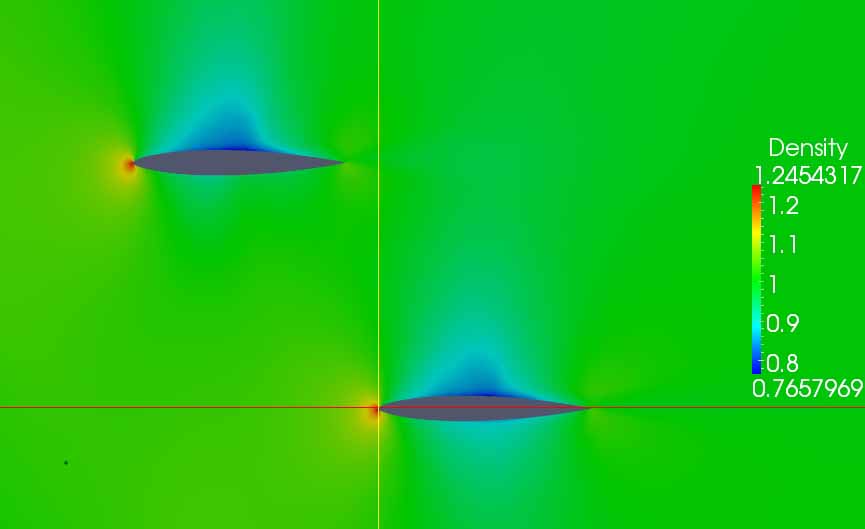
CFD Modeling of Airfoils
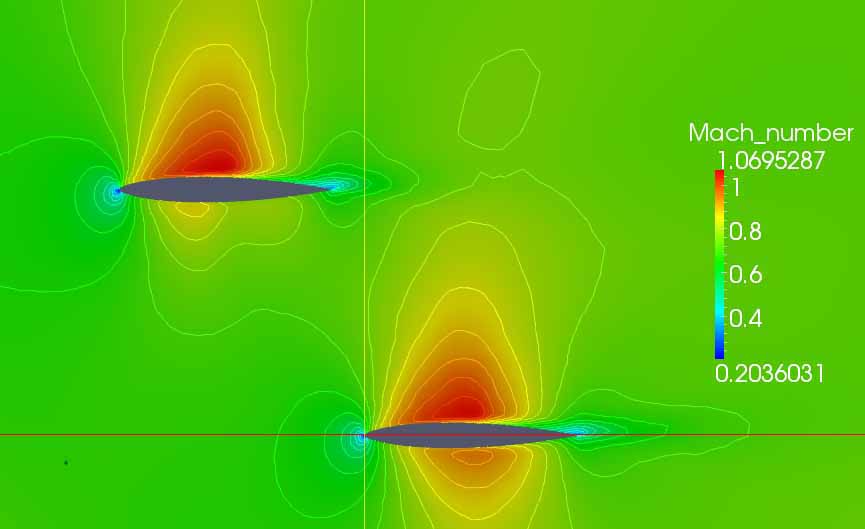
Two adjacent airfoils are modeled with upwind algorithm. The conservation equations of mass, momentum and energy for compressible air are solved simultaneously with Matlab. The shock wave can be well captured and the steady-state velocity, density and pressure fields are computed.